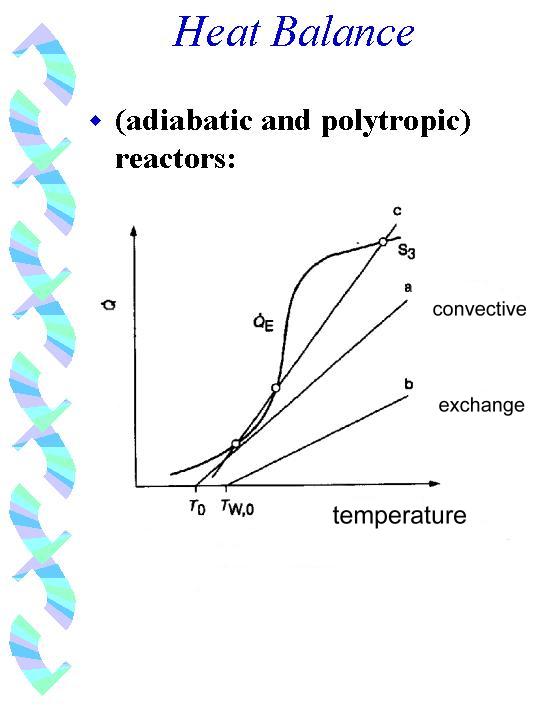
Let us take a CSTR (running stationary), that can be kept adiabatic for the first. As we know 'produces' the (suppose exothermal) reaction with mounting values of conversion mounting quantities of heat (adiabatic temperature rise) and as a consequence mounting temperatures in the stationary vessel. If you plot the values of the heat produced per time from the chemical reaction versus the temperature, you get a S-shaped curve (for an exothermal reaction) (REM: the S-shape comes from the coupling of heat and material balance by the Arrhenius equation):

As this heat has to 'go somewhere' - and in a stable operation mode exactly in a way that the produced and removed heat are equal - you have to search where you can find 'stable' points of intersection of the S-shaped heat production curve and the 'curve' for the 'removing process', - take curve 'c' as an example, without knowing what it represents. These processes might be: the convective flow for an adiabatic CSTR and the convective flow and the heat exchange process for a polytropic CSTR. Both processes exhibit linear functions of dQ/dt versus Temperature (see general heat balance). Therefore curve 'a' could be the heat-removing-curve for some special convective flow. As you realize, 'a' has no intersection with the heat production curve: this convective flow can not 'stabilize' the reactor thermally. Let us take curve 'c' now as a special convective flow. Here we have 3 intersections. The point in the middle of the S has a special feature: when you leave the intersection point in an incremental manner, you will 'rush' either upward on the route of the heat production curve or downward 'along' the 'heat removing curve', - and in both cases 'so long' till you have reached one of the two other intersection points. We call this an 'instable status' of the heat balance or instable operation mode, whereas the other two values are 'stable'. Now a last 'contemplation': let us suppose that the convective flow represented by curve 'a' can not stabilize our adiabatic reactor, as already realized, - what to do then? When the convective flow should not be changed, you could only change its 'input temperature' or you give up the adiabatic operation mode and add a heat exchange equipment. In this polytropic operation mode 'c' as sum of 'a' and 'b' (heat exchange term!) will work in the right manner.
You should be confirmed now in the 'stability matter' and you should also know the alternative visualization in the conversion/temperature plot, ... or ?
take your browser back for previous text or: